An Area Model Can Be Used To Find These
Juapaving
Apr 04, 2025 · 6 min read
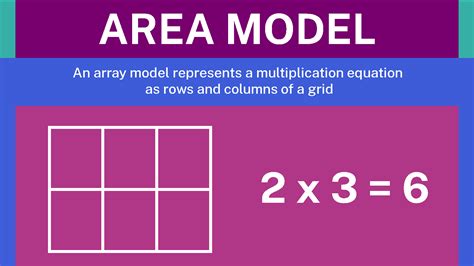
Table of Contents
An Area Model Can Be Used to Find These: Exploring its Applications in Mathematics
The area model, a powerful visual tool in mathematics, extends far beyond simply calculating the area of rectangles. It offers a versatile approach to understanding and solving a wide range of mathematical problems, from basic multiplication and division to more advanced concepts like factoring polynomials and solving systems of equations. This article delves deep into the diverse applications of the area model, showcasing its versatility and effectiveness in various mathematical contexts. We will explore how this seemingly simple visual representation unlocks a deeper understanding of fundamental mathematical principles.
Understanding the Area Model: A Foundation for Deeper Learning
Before we delve into its applications, let's establish a firm understanding of what the area model is. Essentially, it's a visual representation of multiplication and related concepts using rectangles. The dimensions of the rectangle represent the numbers being multiplied, and the area of the rectangle represents the product. For example, to multiply 12 by 15, we can represent 12 as (10 + 2) and 15 as (10 + 5). This breaks down the multiplication into smaller, more manageable parts.
The rectangle is then divided into smaller rectangles, representing the partial products: 10 x 10, 10 x 5, 2 x 10, and 2 x 5. By calculating the area of each smaller rectangle and summing them up, we obtain the total area, which is the product of 12 and 15 (180).
Visualizing Multiplication: A Concrete Approach
The beauty of the area model lies in its visual nature. It provides a concrete representation of abstract mathematical concepts, making it easier for learners, especially younger students, to grasp the underlying principles. This visual approach fosters a deeper understanding than rote memorization of multiplication tables alone. By seeing the components of the multiplication process laid out visually, students can connect the abstract concept to a tangible representation. This concrete understanding forms a strong foundation for more advanced mathematical concepts.
Beyond Basic Multiplication: Expanding the Applications
While its primary use is in multiplication, the area model's applications extend far beyond this basic operation. Its versatility allows it to be applied to a wide range of mathematical problems, including:
Advanced Applications of the Area Model
1. Factoring Quadratic Expressions
One of the most significant applications of the area model is in factoring quadratic expressions. Consider the quadratic expression x² + 5x + 6. We can represent this expression as a rectangle whose area is x² + 5x + 6. By strategically dividing the rectangle into smaller parts based on the coefficients of the terms, we can find its dimensions, which ultimately represent the factors of the quadratic.
This method provides a visual and intuitive way to factor quadratic expressions, making the process less abstract and more accessible. The area model helps students understand the connection between the factors and the original expression, promoting a deeper understanding of the factoring process.
Example: To factor x² + 5x + 6, we can arrange the terms within a rectangle, representing the area. We find two numbers that add up to 5 (the coefficient of x) and multiply to 6 (the constant term). These numbers are 2 and 3. The dimensions of the rectangle would then be (x + 2) and (x + 3), representing the factors of the quadratic expression.
2. Solving Systems of Linear Equations
The area model can also be adapted to solve systems of linear equations. By representing the equations graphically as lines within a coordinate plane, the point of intersection represents the solution to the system. The area of the region enclosed by the lines and the axes can be analyzed to understand the relationship between the equations. While not a direct application of the area model like in multiplication or factoring, the concept of area and spatial reasoning contribute to the understanding of these systems.
3. Understanding Distributive Property
The area model provides a clear visual demonstration of the distributive property. The distributive property states that a(b + c) = ab + ac. When visualized using the area model, the rectangle representing a(b + c) is split into two smaller rectangles with areas ab and ac, clearly illustrating the equivalence. This visual proof makes the abstract concept of the distributive property more concrete and easier to understand.
4. Working with Fractions and Decimals
The area model is equally effective when dealing with fractions and decimals. For example, multiplying fractions like 1/2 by 2/3 can be visually represented using an area model, where the rectangle is divided into appropriate parts to show the product. Similarly, multiplying decimals can be easily represented using the area model by breaking down the decimals into their respective place values. This visual representation can make working with fractions and decimals less daunting for students.
5. Exploring Algebraic Concepts
The power of the area model lies in its ability to represent abstract algebraic concepts visually. It simplifies complex problems, allowing students to manipulate and understand algebraic expressions with greater ease. The area model can be used to solve problems involving polynomials, expanding binomials, and exploring various relationships between variables. This visual representation fosters a deeper understanding of algebraic principles and enhances problem-solving skills.
Benefits of Using the Area Model in Education
The area model offers several crucial benefits in mathematics education:
- Visual Learning: Its visual nature caters to visual learners, making abstract concepts more accessible.
- Concrete Understanding: It provides a concrete representation of abstract mathematical concepts, bridging the gap between the abstract and the tangible.
- Enhanced Problem-Solving Skills: It helps students develop strong problem-solving skills by providing a structured approach to solving problems.
- Improved Conceptual Understanding: It encourages deeper understanding of underlying mathematical principles rather than rote memorization.
- Versatile Application: Its versatility allows its application across various mathematical domains, fostering a cohesive understanding of mathematical concepts.
- Differentiated Instruction: It can be adapted to suit diverse learning styles and abilities, making it a valuable tool for differentiated instruction.
Conclusion: The Enduring Power of Visual Representation
The area model serves as a powerful tool for understanding and solving a wide array of mathematical problems. Its versatility, coupled with its visual nature, makes it an invaluable resource for educators and students alike. From basic multiplication to advanced algebraic concepts, the area model provides a concrete and intuitive approach to learning mathematics, fostering a deeper understanding and improved problem-solving skills. By embracing this visual approach, we can empower students to engage more effectively with mathematical concepts and develop a strong foundation for future learning. Its ability to connect abstract ideas with tangible visual representations ensures its continued relevance and importance in mathematics education. The area model is not just a method; it’s a pathway to a more intuitive and profound understanding of mathematics. Its enduring power lies in its ability to translate abstract mathematical concepts into visually accessible representations, making learning more engaging and effective for students of all levels.
Latest Posts
Latest Posts
-
What Energy Transfer Takes Place In A Generator
Apr 04, 2025
-
A Triplet Of Mrna Is Called A
Apr 04, 2025
-
According To Bohr Model How Do Electrons Move In Atoms
Apr 04, 2025
-
What Is The Current Through 10 Volt Battery
Apr 04, 2025
-
Cam Plants Keep Stomata Closed In The Daytime
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about An Area Model Can Be Used To Find These . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
