7 Out Of 20 As A Percentage
Juapaving
May 11, 2025 · 4 min read
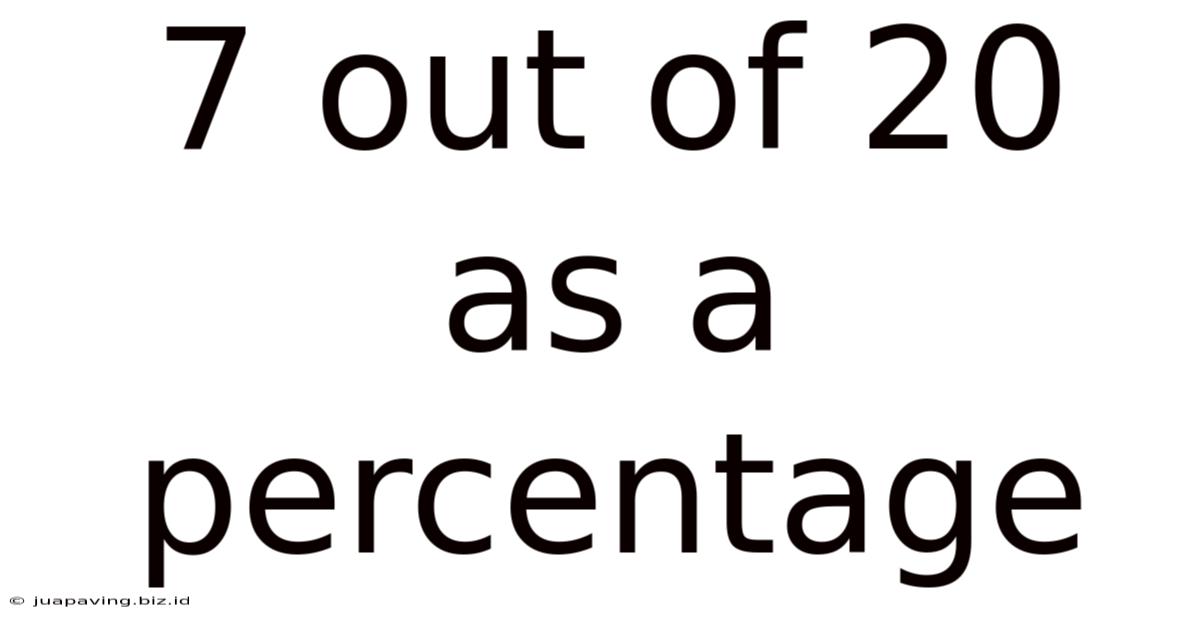
Table of Contents
7 Out of 20 as a Percentage: A Comprehensive Guide
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating discounts and tax rates to analyzing data and comprehending statistics. This article delves into the specific calculation of 7 out of 20 as a percentage, providing a clear, step-by-step process and exploring the broader context of percentage calculations. We will also touch upon practical applications and common misconceptions.
Understanding Percentages: The Basics
Before diving into the specifics of 7 out of 20, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a portion of 100. The term "percent" literally means "per hundred." Therefore, 25% means 25 out of 100, or 25/100, which simplifies to 1/4.
Calculating 7 Out of 20 as a Percentage: The Method
The core principle involves converting the fraction 7/20 into its equivalent percentage. Here's the detailed breakdown:
Step 1: Set up the Fraction
The problem presents itself as a fraction: 7 out of 20 can be written as 7/20.
Step 2: Convert the Fraction to a Decimal
To convert a fraction to a decimal, divide the numerator (the top number) by the denominator (the bottom number):
7 ÷ 20 = 0.35
Step 3: Convert the Decimal to a Percentage
Multiply the decimal by 100 to express it as a percentage:
0.35 × 100 = 35%
Therefore, 7 out of 20 is equal to 35%.
Alternative Methods: Proportions and Cross-Multiplication
While the above method is straightforward, alternative methods can be helpful, especially when dealing with more complex scenarios. One such method utilizes proportions.
We can set up a proportion:
7/20 = x/100
Where 'x' represents the percentage we want to find.
Using cross-multiplication:
20x = 700
Solving for 'x':
x = 700 ÷ 20 = 35
Therefore, x = 35%, confirming our previous calculation.
Practical Applications of Percentage Calculations: Real-world Examples
The ability to calculate percentages is crucial in numerous real-world contexts:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For example, understanding a 10% discount on a $100 item means you save $10.
-
Statistics and Data Analysis: Percentages are essential for representing data in a clear and concise manner. In surveys, for instance, results are often presented as percentages to show the proportion of respondents who chose a particular option. Imagine a survey where 7 out of 20 people preferred a certain brand of coffee; this translates to a 35% preference.
-
Academic Performance: Grading systems often use percentages to represent a student's performance on exams and assignments. A score of 7 out of 20 on a test might indicate a 35% grade.
-
Sales and Marketing: Tracking sales figures, conversion rates, and market share frequently involves calculating and interpreting percentages. A company might analyze that 35% of website visitors make a purchase.
-
Everyday Life: Understanding percentages helps in comparing prices, evaluating deals, and making informed decisions about purchases. This skill is essential for managing personal finances effectively.
Common Misconceptions About Percentages
Several common misconceptions can lead to errors in percentage calculations:
-
Confusing Percentage with Fraction: While percentages are derived from fractions, they aren't interchangeable in all contexts. A percentage always refers to a portion of 100, while a fraction represents a part of a whole.
-
Incorrectly Adding or Subtracting Percentages: Percentages cannot be directly added or subtracted unless they are based on the same whole. For instance, you can't simply add a 10% increase followed by a 20% decrease to say there was a net 10% decrease. The calculation needs to account for the change in the base value after each percentage change.
-
Misunderstanding Percentage Increase/Decrease: Calculating a percentage increase or decrease requires careful attention to the base value. A 10% increase on $100 is $110, but a 10% decrease from $110 is not back to $100. The base value changes.
Advanced Percentage Calculations: Beyond the Basics
While 7 out of 20 is a relatively simple calculation, more complex percentage problems may arise. These can involve calculating percentage changes, finding the original value after a percentage increase or decrease, or working with compound interest. Mastering these requires a deeper understanding of the underlying principles and often involves using algebraic equations.
Conclusion: Mastering Percentages for Success
The ability to calculate percentages, even simple ones like 7 out of 20, is a fundamental skill with widespread applications. This article has provided a comprehensive explanation of how to calculate 7 out of 20 as a percentage (35%), explored various methods for solving similar problems, and highlighted the importance of understanding percentages in various aspects of life. By mastering percentage calculations, you equip yourself with a valuable tool for navigating financial decisions, analyzing data, and making informed choices in numerous situations. Remember to always double-check your work and be aware of common misconceptions to ensure accuracy. With practice, you’ll become proficient in applying this essential skill.
Latest Posts
Latest Posts
-
On What Organelle Does This Process Occur
May 12, 2025
-
Number Of Vertices On A Rectangular Prism
May 12, 2025
-
Surface Integral Of A Vector Field
May 12, 2025
-
The Lines On A Solubility Graph Indicate Where A Solution
May 12, 2025
-
What Is The Reciprocal Of 4 5
May 12, 2025
Related Post
Thank you for visiting our website which covers about 7 Out Of 20 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.