6 Of 8 Is What Percent
Juapaving
May 11, 2025 · 4 min read
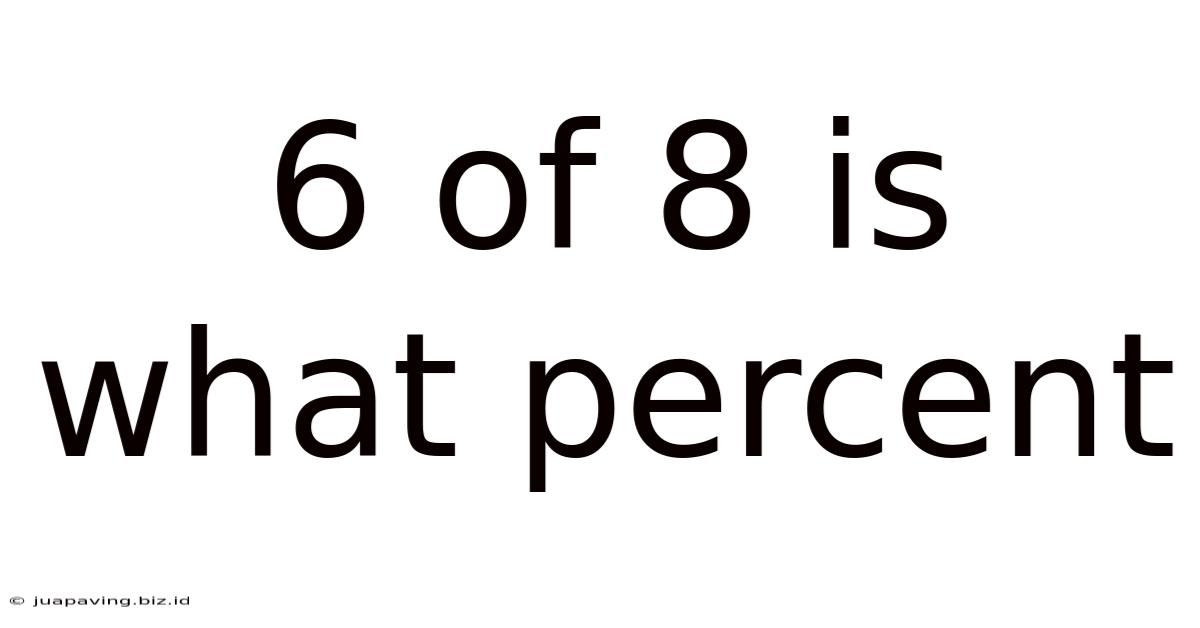
Table of Contents
6 out of 8 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous aspects of life, from calculating discounts and taxes to comprehending statistical data and analyzing financial reports. This comprehensive guide will delve into the question, "6 out of 8 is what percent?", providing a step-by-step explanation, various calculation methods, and practical applications. We'll explore the core concepts, tackle similar problems, and even touch upon advanced percentage applications.
Understanding the Fundamentals of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred." Therefore, 50% means 50 out of 100, which is equivalent to ½ or 0.5. To express a fraction or ratio as a percentage, we need to convert it to an equivalent fraction with a denominator of 100.
Calculating "6 out of 8" as a Percentage: Three Methods
There are several ways to calculate what percentage 6 out of 8 represents. Let's explore three common methods:
Method 1: Using the Fraction Method
This method is straightforward and directly addresses the core concept of percentages.
-
Express the ratio as a fraction: The ratio "6 out of 8" is written as the fraction 6/8.
-
Simplify the fraction (optional but recommended): Both 6 and 8 are divisible by 2, so we can simplify the fraction to 3/4. Simplifying makes the calculation easier.
-
Convert the fraction to a percentage: To convert a fraction to a percentage, we multiply the fraction by 100%.
(3/4) * 100% = 75%
Therefore, 6 out of 8 is 75%.
Method 2: Using Decimal Conversion
This method involves converting the fraction to a decimal first, then converting the decimal to a percentage.
-
Express the ratio as a fraction: Again, we start with the fraction 6/8.
-
Convert the fraction to a decimal: Divide the numerator (6) by the denominator (8): 6 ÷ 8 = 0.75
-
Convert the decimal to a percentage: Multiply the decimal by 100%: 0.75 * 100% = 75%
This method confirms that 6 out of 8 is 75%.
Method 3: Using Proportion
This method uses the concept of proportions to solve the problem. We set up a proportion where x represents the percentage we're looking for:
6/8 = x/100
To solve for x, we cross-multiply:
8x = 600
Then, divide both sides by 8:
x = 600/8 = 75
Therefore, x = 75%, confirming once again that 6 out of 8 is 75%.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in numerous real-world scenarios. Here are a few examples:
-
Grade Calculations: If you answered 6 questions correctly out of 8 on a quiz, your score is 75%.
-
Sales and Discounts: A store offering a 25% discount on an item means you'll pay 75% of the original price. If the original price is $80, you'll pay $60 (75% of $80).
-
Financial Analysis: Percentage change is used to track increases or decreases in stock prices, profits, or expenses. For example, if a company's profit increased from $6 million to $8 million, the percentage increase is 33.33%.
-
Statistical Data: Percentages are frequently used to represent data in charts, graphs, and reports. For example, a survey might show that 75% of respondents prefer a particular product.
Solving Similar Percentage Problems
Let's practice with a few more examples to solidify your understanding:
Example 1: What percentage is 4 out of 10?
Using the fraction method: 4/10 = 2/5 = (2/5) * 100% = 40%
Example 2: What percentage is 12 out of 16?
Using the fraction method: 12/16 = 3/4 = (3/4) * 100% = 75%
Example 3: What percentage is 15 out of 25?
Using the fraction method: 15/25 = 3/5 = (3/5) * 100% = 60%
Advanced Percentage Applications
Beyond basic percentage calculations, there are more complex applications:
-
Percentage Increase/Decrease: These calculations are crucial for analyzing changes over time. The formula for percentage increase is: [(New Value - Old Value) / Old Value] * 100%. The formula for percentage decrease is similar but with the difference being negative.
-
Compound Interest: Compound interest involves earning interest on both the principal amount and accumulated interest. The formula for compound interest is: A = P (1 + r/n)^(nt), where A is the final amount, P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time in years.
-
Taxes and Discounts: Calculating sales tax or discounts often involves multiple percentage calculations. For example, you might need to calculate a 6% sales tax on an item that is already discounted by 20%.
Conclusion: Mastering Percentage Calculations
Understanding percentages is a versatile skill with wide-ranging applications. By mastering the various methods of calculation, from the straightforward fraction method to the more advanced applications, you'll be well-equipped to tackle various percentage-related problems in academic, professional, and everyday life. Remember to practice regularly, and don't hesitate to break down complex problems into smaller, manageable steps. This will help build confidence and fluency in your ability to work with percentages effectively. The ability to quickly and accurately calculate percentages is a valuable asset in many areas of life, from personal finance to professional success.
Latest Posts
Latest Posts
-
Which Of The Following Is Not A Business Transaction
May 12, 2025
-
Who Is The Father Of Humanism
May 12, 2025
-
A Group Of Cows Are Called
May 12, 2025
-
What Is The Lowest Common Multiple Of 6 And 21
May 12, 2025
-
What Is A Node In Waves
May 12, 2025
Related Post
Thank you for visiting our website which covers about 6 Of 8 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.