5 Is What Percent Of 8
Juapaving
Apr 05, 2025 · 5 min read
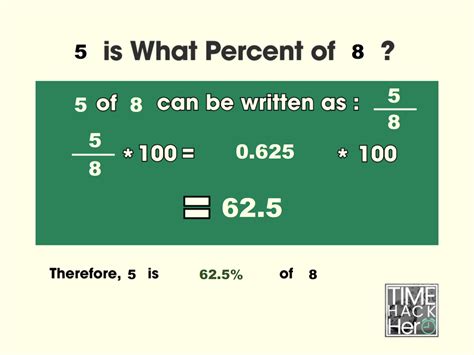
Table of Contents
5 is What Percent of 8? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding financial reports. This article will delve deep into the question, "5 is what percent of 8?", providing not only the answer but also a comprehensive explanation of the underlying principles and practical applications of percentage calculations. We'll explore different methods for solving this problem, discuss common pitfalls, and offer valuable tips for mastering percentage calculations. By the end, you'll not only know the answer but also possess a robust understanding of percentages and their practical uses.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred." For instance, 25% means 25 out of 100, which can also be written as the fraction 25/100 or the decimal 0.25. Percentages are used extensively because they provide a standardized and easily understandable way to compare proportions and make relative comparisons.
Method 1: Using the Proportion Method
This is arguably the most intuitive method for solving percentage problems. We can set up a proportion to solve "5 is what percent of 8?"
The proportion will look like this:
5/8 = x/100
Where:
- 5 represents the part.
- 8 represents the whole.
- x represents the unknown percentage we're trying to find.
- 100 represents the total percentage (100%).
To solve for x, we cross-multiply:
8 * x = 5 * 100
8x = 500
x = 500 / 8
x = 62.5
Therefore, 5 is 62.5% of 8.
Step-by-Step Breakdown:
- Identify the parts: Clearly define the part (5) and the whole (8).
- Set up the proportion: Create a proportion relating the part to the whole and the unknown percentage to 100%.
- Cross-multiply: Multiply the numerator of one fraction by the denominator of the other.
- Solve for x: Isolate the variable 'x' by dividing both sides of the equation by the coefficient of 'x'.
- Express the answer: State the answer as a percentage.
Method 2: Using the Decimal Method
This method involves converting the fraction into a decimal and then multiplying by 100 to express it as a percentage.
- Express as a fraction: Write the problem as a fraction: 5/8
- Convert to decimal: Divide the numerator (5) by the denominator (8): 5 ÷ 8 = 0.625
- Convert to percentage: Multiply the decimal by 100: 0.625 * 100 = 62.5%
Therefore, 5 is 62.5% of 8.
Step-by-Step Breakdown:
- Fraction representation: Represent the problem as a fraction showing the part over the whole.
- Decimal conversion: Use a calculator or long division to convert the fraction to its decimal equivalent.
- Percentage conversion: Multiply the decimal by 100 to express the result as a percentage.
Method 3: Using the Formula Method
A more direct formula approach can be used:
Percentage = (Part / Whole) * 100
In this case:
Percentage = (5 / 8) * 100 = 62.5%
This formula encapsulates the essence of the previous methods in a concise form.
Step-by-Step Breakdown:
- Identify parts: Determine the part (5) and the whole (8).
- Substitute values: Substitute these values into the formula.
- Calculate: Perform the calculation to find the percentage.
Practical Applications of Percentage Calculations
The ability to calculate percentages is crucial in numerous real-world scenarios:
-
Financial Calculations: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. For example, understanding the interest rate on a loan or the discount on a sale item requires a solid grasp of percentages.
-
Data Analysis: Percentages are essential for interpreting data and making comparisons. For instance, analyzing survey results, understanding market shares, or comparing performance metrics often involves calculating and interpreting percentages. Analyzing sales figures, for example, frequently requires calculating percentage increases or decreases in sales over time.
-
Scientific Applications: Percentages are used to express concentrations, error margins, and statistical probabilities. In scientific research, expressing results and variations as percentages aids in clarity and comparison.
-
Everyday Life: We encounter percentages in various daily situations, such as calculating tips in restaurants, determining sale prices, and understanding nutritional information on food labels. Understanding percentages helps in making informed decisions in everyday life.
Common Pitfalls to Avoid
Several common mistakes can lead to inaccurate percentage calculations. Being aware of these pitfalls can help improve accuracy:
-
Incorrectly Identifying the Part and the Whole: Carefully identify which number represents the part and which represents the whole. Mixing these up will lead to an incorrect answer.
-
Errors in Decimal Calculations: Pay close attention to decimal points when converting fractions to decimals and vice versa. Even a minor decimal error can significantly impact the final result.
-
Forgetting to Multiply by 100: When converting a decimal to a percentage, always remember to multiply the decimal by 100. This crucial step transforms the decimal into its percentage equivalent.
Advanced Percentage Problems
While the problem "5 is what percent of 8?" is a relatively straightforward percentage calculation, understanding the underlying principles allows you to tackle more complex percentage problems. These might involve finding the percentage increase or decrease between two numbers, calculating percentages of percentages, or dealing with more complex scenarios involving multiple percentages.
Conclusion
The answer to "5 is what percent of 8?" is 62.5%. This article has explored several methods for calculating percentages, highlighting the importance of understanding the underlying principles and demonstrating their practical applications in various contexts. By mastering percentage calculations, you enhance your ability to analyze data, make informed decisions, and navigate various aspects of life more effectively. Remember to practice regularly, paying close attention to the steps involved, to build confidence and proficiency in this essential mathematical skill. Understanding percentages is not just about solving mathematical problems; it's about gaining a valuable tool for understanding and interpreting the world around you.
Latest Posts
Latest Posts
-
Examples Of Abiotic Factors And Biotic Factors
Apr 06, 2025
-
For Every Action There Is An Equal Opposite Reaction
Apr 06, 2025
-
A Comparison Of Two Similar Quantities Using Division
Apr 06, 2025
-
Round 0 234 To The Nearest Hundredth
Apr 06, 2025
-
An Inch Is What Fraction Of A Yard
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about 5 Is What Percent Of 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
