35 9 As A Mixed Number
Juapaving
May 10, 2025 · 5 min read
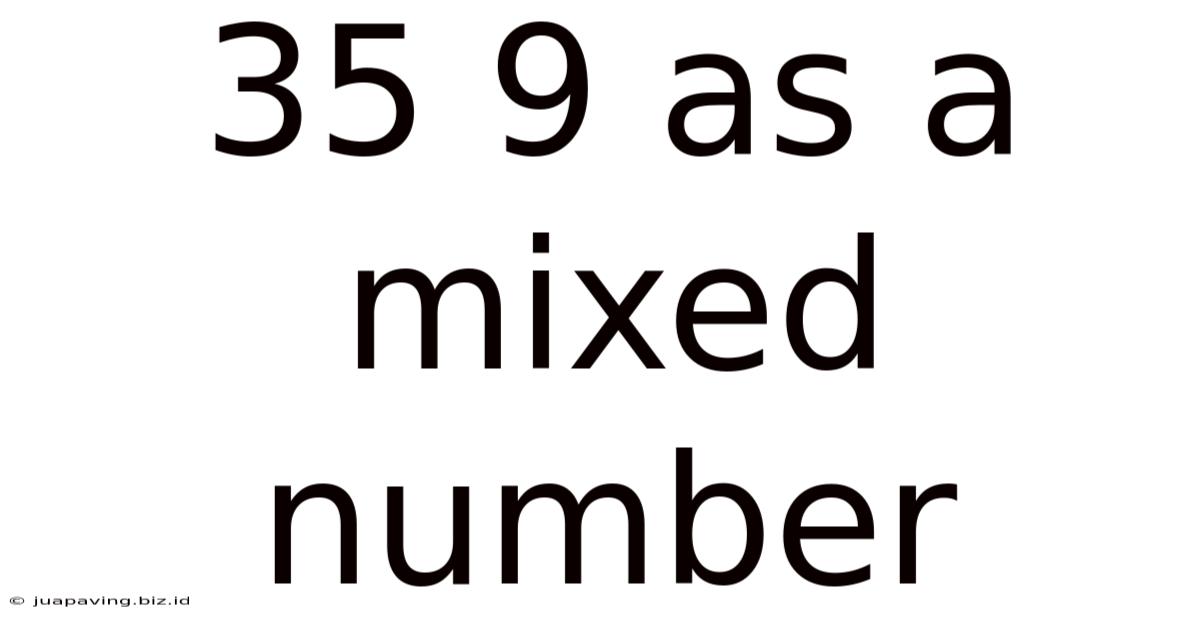
Table of Contents
35/9 as a Mixed Number: A Comprehensive Guide
Understanding fractions and how to convert them into mixed numbers is a fundamental skill in mathematics. This comprehensive guide will delve into the process of converting the improper fraction 35/9 into a mixed number, explaining the underlying concepts and providing practical examples. We'll explore different methods and address common misconceptions, ensuring a thorough understanding of this essential mathematical operation. This article is optimized for search engines, incorporating relevant keywords and semantic variations to improve its visibility and ranking.
What is a Mixed Number?
A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 2 ¾ is a mixed number; it represents two whole units and three-quarters of another unit. Conversely, an improper fraction is a fraction where the numerator is greater than or equal to the denominator, such as 11/4. Converting improper fractions to mixed numbers makes it easier to visualize and understand the quantity represented.
Converting 35/9 to a Mixed Number: Step-by-Step
The process of converting 35/9 into a mixed number involves dividing the numerator (35) by the denominator (9). Here's a detailed breakdown:
Step 1: Perform the Division
Divide 35 by 9:
35 ÷ 9 = 3 with a remainder of 8
Step 2: Identify the Whole Number and Remainder
The quotient (the result of the division) becomes the whole number part of the mixed number. In this case, the quotient is 3. The remainder (the number left over after the division) becomes the numerator of the fractional part. The remainder is 8.
Step 3: Construct the Mixed Number
The denominator of the fractional part remains the same as the denominator of the original improper fraction. Therefore, the denominator remains 9.
Combining the whole number and the fraction, we get the mixed number:
3 ⁸⁄₉
Therefore, 35/9 expressed as a mixed number is 3 ⁸⁄₉. This means there are three whole units and eight-ninths of another unit.
Alternative Methods for Conversion
While the long division method is the most common approach, other methods can be used to convert improper fractions to mixed numbers. Let's explore a couple of them:
Method 2: Repeated Subtraction
This method involves repeatedly subtracting the denominator from the numerator until the result is less than the denominator. The number of times you subtract the denominator represents the whole number part of the mixed number, and the remaining value becomes the numerator of the fractional part.
Let's apply this to 35/9:
- 35 - 9 = 26
- 26 - 9 = 17
- 17 - 9 = 8
We subtracted 9 three times before the remaining value (8) was less than 9. Therefore, the whole number is 3, and the remainder (8) forms the numerator of the fraction. The denominator remains 9. This gives us the mixed number 3 ⁸⁄₉.
Method 3: Using Multiplication and Subtraction (Less efficient, but illustrative)
This method is less efficient than direct division, but it helps visualize the concept. You find how many times the denominator fits into the numerator without exceeding it.
We know that 9 x 3 = 27, which is close to 35 but less than it. Then, we find the difference between 35 and 27: 35 - 27 = 8. This difference is the remainder, which becomes the numerator of the fraction. The whole number is 3 (from 9 x 3), and the denominator remains 9. This again results in 3 ⁸⁄₉.
Visual Representation of 35/9
Visualizing the fraction can aid in understanding the concept. Imagine 35 identical objects. If you group them into sets of 9, you'll have 3 complete sets (3 whole units) and 8 objects remaining (8/9 of a set). This visually reinforces the mixed number representation of 3 ⁸⁄₉.
Importance of Understanding Mixed Numbers
Converting improper fractions to mixed numbers is crucial for several reasons:
- Improved Comprehension: Mixed numbers provide a clearer and more intuitive representation of quantities, especially in real-world applications. For instance, it's easier to understand 3 ⁸⁄₉ pizzas than 35/9 pizzas.
- Simplification of Calculations: Mixed numbers often simplify arithmetic operations, especially addition and subtraction of fractions.
- Problem Solving: Many mathematical problems, particularly those involving measurements and quantities, require working with mixed numbers.
Common Mistakes to Avoid
When converting improper fractions to mixed numbers, it's important to avoid these common errors:
- Incorrect Division: Ensure you perform the division correctly. A simple mistake in division will lead to an incorrect mixed number.
- Forgetting the Remainder: The remainder is an essential component of the mixed number; neglecting it will result in an inaccurate representation.
- Incorrect Placement of Numbers: Pay close attention to the placement of the whole number and the fractional part in the mixed number.
Practical Applications of Mixed Numbers
Mixed numbers are widely used in various contexts:
- Cooking and Baking: Recipe measurements frequently utilize mixed numbers (e.g., 2 ½ cups of flour).
- Construction and Engineering: Measurements in construction and engineering often involve mixed numbers for precise calculations.
- Everyday Life: Many everyday scenarios require dealing with fractional parts, which are best represented using mixed numbers for clarity.
Conclusion
Converting the improper fraction 35/9 to the mixed number 3 ⁸⁄₉ is a straightforward process that involves dividing the numerator by the denominator. Understanding this conversion is vital for various mathematical applications and helps in simplifying calculations and improving comprehension. By mastering this skill, you enhance your problem-solving capabilities and improve your overall understanding of fractions. Remember to practice regularly to reinforce your understanding and avoid common mistakes. This comprehensive guide provides a solid foundation for understanding this essential mathematical concept. Now you should be well-equipped to tackle similar conversions with confidence!
Latest Posts
Latest Posts
-
Write The Formula For Hydroiodic Acid
May 10, 2025
-
Is A Colloid A Homogeneous Mixture
May 10, 2025
-
What Is The Boiling Point Of Kelvin
May 10, 2025
-
What Happens To Acceleration When Force Is Doubled
May 10, 2025
-
Which Of The Following Is The Smallest In Size
May 10, 2025
Related Post
Thank you for visiting our website which covers about 35 9 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.