3 Out Of 20 As A Percentage
Juapaving
May 10, 2025 · 4 min read
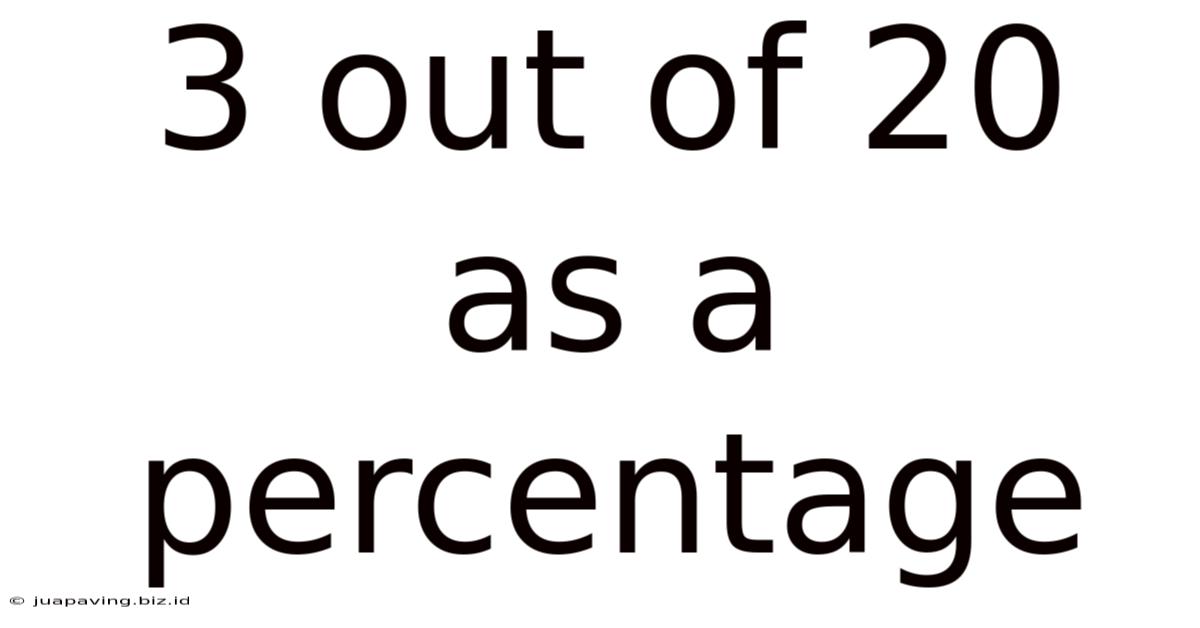
Table of Contents
3 out of 20 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from everyday budgeting to complex statistical analysis. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and excelling in various academic and professional settings. This in-depth guide will focus specifically on calculating "3 out of 20 as a percentage," explaining the process step-by-step and exploring its applications. We’ll also delve into the broader context of percentage calculations and offer practical tips for mastering this essential skill.
Understanding Percentages
Before diving into the specific calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Understanding this basic principle is crucial. Any fraction can be converted into a percentage by expressing the fraction with a denominator of 100. However, there are more efficient methods, which we'll explore below.
Calculating 3 out of 20 as a Percentage: Step-by-Step
To calculate "3 out of 20" as a percentage, we follow these straightforward steps:
Step 1: Express as a Fraction
First, we express the given information as a fraction: 3/20. This represents 3 parts out of a total of 20 parts.
Step 2: Convert the Fraction to a Decimal
To convert the fraction 3/20 into a decimal, we divide the numerator (3) by the denominator (20):
3 ÷ 20 = 0.15
Step 3: Convert the Decimal to a Percentage
Finally, to convert the decimal 0.15 into a percentage, we multiply by 100 and add the "%" symbol:
0.15 x 100 = 15%
Therefore, 3 out of 20 is equal to 15%.
Alternative Calculation Methods
While the above method is the most straightforward, there are alternative approaches to arrive at the same result.
Method 1: Finding an Equivalent Fraction with a Denominator of 100
We can manipulate the fraction 3/20 to find an equivalent fraction with a denominator of 100. Since 20 x 5 = 100, we multiply both the numerator and denominator by 5:
(3 x 5) / (20 x 5) = 15/100
This directly gives us the percentage, which is 15%.
Method 2: Using Proportions
We can also solve this using proportions. We set up a proportion:
3/20 = x/100
To solve for x (the percentage), we cross-multiply:
20x = 300
x = 300/20 = 15
Therefore, x = 15%.
Practical Applications of Percentage Calculations
The ability to calculate percentages is invaluable in a wide range of scenarios:
-
Finance: Calculating interest rates, discounts, tax rates, profit margins, and investment returns all involve percentage calculations. Understanding how much interest you're paying on a loan or how much discount you're receiving on a purchase relies directly on these skills.
-
Statistics: Percentages are fundamental in data analysis and interpretation. Representing data as percentages makes it easier to compare and understand different datasets. For instance, understanding the percentage of students who passed an exam or the percentage of people who prefer a specific product requires a solid grasp of percentage calculations.
-
Science: Percentage calculations are used extensively in scientific experiments and data analysis. For example, expressing the concentration of a solution or the percentage error in an experiment requires this skill.
-
Everyday Life: Percentages are ubiquitous in our daily lives. Understanding sales discounts, calculating tips, determining nutritional values from food labels, and interpreting survey results all involve percentage calculations.
Beyond 3 out of 20: Mastering Percentage Calculations
While we've focused on "3 out of 20," the principles we've discussed are applicable to any percentage calculation. To further enhance your skills:
-
Practice Regularly: Consistent practice is key to mastering any mathematical concept. Try calculating various fractions as percentages to build fluency and confidence.
-
Use Different Methods: Explore different methods, such as the ones described above, to reinforce your understanding and find the approach that best suits your style.
-
Utilize Online Calculators (Sparingly): Online calculators can be helpful for checking your work, but it's crucial to understand the underlying principles. Over-reliance on calculators can hinder your ability to perform these calculations independently.
-
Relate Percentages to Real-World Situations: Connect percentage calculations to real-world scenarios to make the learning process more engaging and memorable.
Troubleshooting Common Mistakes
-
Incorrect Fraction Formation: Ensure you correctly identify the numerator (the part) and the denominator (the whole) before starting the calculation.
-
Errors in Decimal Conversion: Pay close attention when dividing the numerator by the denominator to avoid decimal errors.
-
Forgetting to Multiply by 100: Remember to multiply the decimal by 100 to express the result as a percentage.
Conclusion
Understanding how to calculate percentages, even simple ones like "3 out of 20," is a crucial skill with broad applications. By mastering the techniques explained in this guide, you will enhance your problem-solving abilities and gain valuable insights into data interpretation in various contexts. Remember, consistent practice and a solid understanding of the underlying principles are essential for achieving proficiency in percentage calculations. This skill will undoubtedly serve you well in both academic and professional pursuits, empowering you to make informed decisions based on numerical data. So, practice consistently, and you’ll be well on your way to mastering percentages!
Latest Posts
Latest Posts
-
Inner Transition Metals On The Periodic Table
May 10, 2025
-
The Term Climacteric Refers To The
May 10, 2025
-
Compare And Contrast Light And Electron Microscopes
May 10, 2025
-
In What Way Is Artificial Selection Different From Natural Selection
May 10, 2025
-
How Are Point Mutations Different Than Frameshiift
May 10, 2025
Related Post
Thank you for visiting our website which covers about 3 Out Of 20 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.