19 2 As A Mixed Number
Juapaving
May 13, 2025 · 4 min read
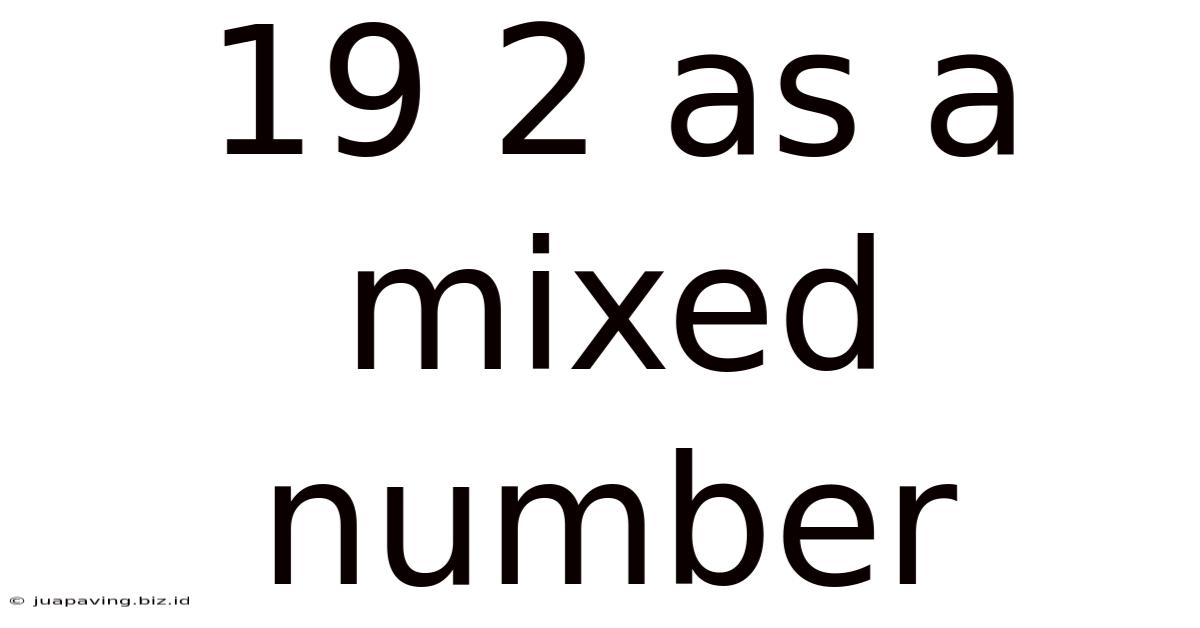
Table of Contents
19/2 as a Mixed Number: A Comprehensive Guide
Understanding fractions and how to convert them into mixed numbers is a fundamental skill in mathematics. This comprehensive guide will delve into the conversion of the improper fraction 19/2 into a mixed number, explaining the process step-by-step and exploring related concepts to solidify your understanding. We'll also touch upon the importance of this conversion in various applications and provide some practical examples.
What is a Mixed Number?
A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1 ½, 3 ¼, and 7 2/5 are all mixed numbers. They represent a quantity that is more than one whole unit but less than the next whole number.
What is an Improper Fraction?
An improper fraction is a fraction where the numerator is greater than or equal to the denominator. Examples include 7/4, 19/2, and 11/11. These fractions represent a quantity equal to or greater than one whole unit. The improper fraction 19/2 is the focus of our discussion today.
Converting 19/2 to a Mixed Number: The Step-by-Step Process
Converting an improper fraction to a mixed number involves dividing the numerator by the denominator. Let's break down the conversion of 19/2:
-
Divide the numerator (19) by the denominator (2): 19 ÷ 2 = 9 with a remainder of 1.
-
The quotient (9) becomes the whole number part of the mixed number. This represents how many whole units are contained in the improper fraction.
-
The remainder (1) becomes the numerator of the fractional part of the mixed number.
-
The denominator remains the same (2).
Therefore, 19/2 as a mixed number is 9 1/2.
This means that 19/2 represents 9 whole units and an additional half unit.
Visualizing the Conversion
Imagine you have 19 pizzas, and you want to divide them equally among 2 people. You can give each person 9 whole pizzas (9 x 2 = 18 pizzas). This leaves you with 1 pizza remaining. You can represent this remaining pizza as 1/2 a pizza per person. Therefore, each person receives 9 1/2 pizzas, visually representing the mixed number 9 1/2.
Why is Converting Improper Fractions Important?
Converting improper fractions to mixed numbers is crucial for several reasons:
-
Easier Interpretation: Mixed numbers are often easier to understand and visualize than improper fractions. It's simpler to grasp the concept of 9 1/2 pizzas than 19/2 pizzas.
-
Real-World Applications: Many real-world scenarios involve quantities that are best represented by mixed numbers. For example, measuring ingredients in a recipe, calculating distances, or representing time.
-
Simplifying Calculations: In some mathematical operations, particularly addition and subtraction, working with mixed numbers can be more straightforward than working with improper fractions.
-
Problem Solving: Many word problems require converting between improper fractions and mixed numbers for efficient problem-solving.
Further Exploration: Converting Mixed Numbers to Improper Fractions
Understanding the reverse process is equally important. To convert a mixed number back into an improper fraction:
-
Multiply the whole number by the denominator: For 9 1/2, this is 9 x 2 = 18.
-
Add the numerator to the result: 18 + 1 = 19.
-
Keep the denominator the same: The denominator remains 2.
Therefore, 9 1/2 converts back to 19/2. This demonstrates the equivalence between the two representations.
Practice Problems: Converting Improper Fractions to Mixed Numbers
Let's solidify your understanding with a few practice problems:
- Convert 23/5 to a mixed number.
- Convert 37/8 to a mixed number.
- Convert 41/6 to a mixed number.
Solutions:
- 23/5 = 4 3/5
- 37/8 = 4 5/8
- 41/6 = 6 5/6
By practicing these conversions, you'll become more proficient in working with fractions and mixed numbers.
The Importance of Fractions in Different Fields
Fractions and the ability to convert between improper fractions and mixed numbers are fundamental across various fields:
-
Baking and Cooking: Recipes often require precise measurements, utilizing fractions and mixed numbers to specify quantities of ingredients.
-
Construction and Engineering: Accurate measurements and calculations are vital in construction and engineering. Understanding fractions ensures precision and avoids errors.
-
Finance: Calculating interest, proportions, and shares frequently involves working with fractions.
-
Science: In scientific experiments and measurements, precise fractions are crucial for accuracy and reproducibility.
Mastering Fractions: A Continuous Journey
The conversion of 19/2 to the mixed number 9 1/2 is a seemingly simple process but represents a crucial stepping stone in mastering fractional arithmetic. Consistent practice and understanding the underlying concepts are key to achieving fluency in working with fractions and mixed numbers. Remember that practice is the key to mastering this skill and applying it effectively across various mathematical contexts. Embrace the challenge, and you'll find that working with fractions becomes increasingly intuitive and rewarding. Don't hesitate to explore more complex fraction problems and seek out additional resources to deepen your understanding. Your mathematical journey is a continuous process of learning and refinement; keep exploring!
Latest Posts
Latest Posts
-
List The First Five Multiples Of 12
May 13, 2025
-
The Genetic Makeup Of An Organism Is Called
May 13, 2025
-
Which Statement Is True About Neuroglial Cells
May 13, 2025
-
How Many Legs On A Octopus
May 13, 2025
-
9 Yards Is How Many Feet
May 13, 2025
Related Post
Thank you for visiting our website which covers about 19 2 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.