18 7 As A Mixed Number
Juapaving
May 13, 2025 · 5 min read
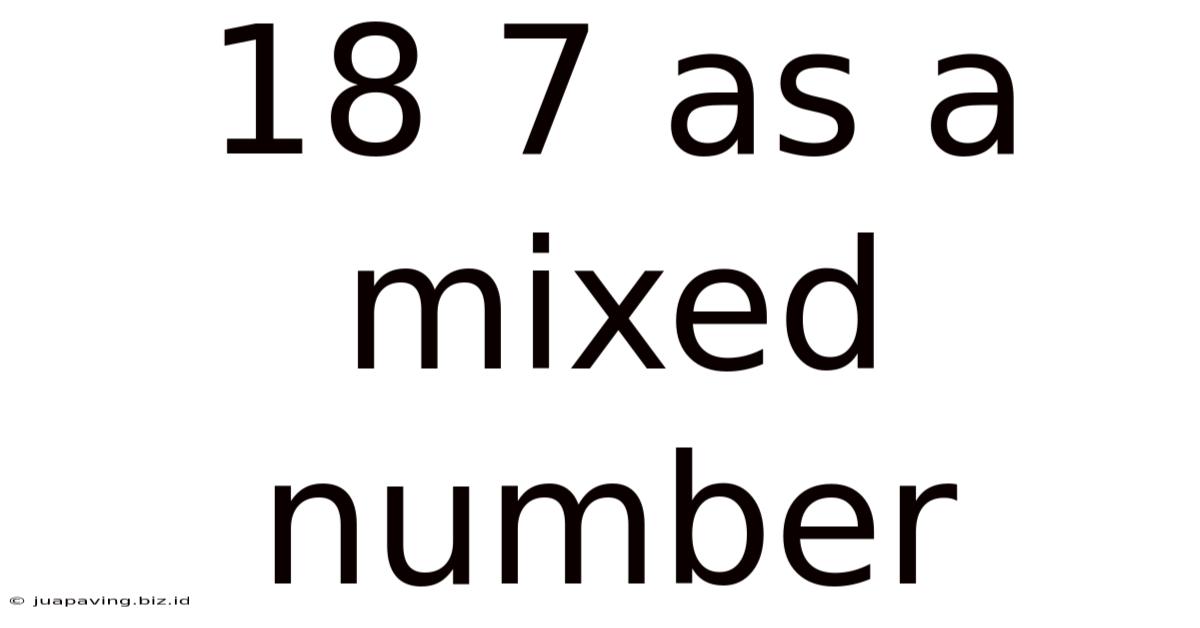
Table of Contents
18/7 as a Mixed Number: A Comprehensive Guide
Converting improper fractions, like 18/7, into mixed numbers is a fundamental skill in arithmetic. This guide provides a thorough explanation of the process, explores different methods, and offers practical examples to solidify your understanding. We'll delve into why this conversion is important and how it applies to various mathematical contexts. By the end, you'll be confident in converting any improper fraction to its mixed number equivalent.
Understanding Improper Fractions and Mixed Numbers
Before diving into the conversion, let's clarify the terms:
-
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). Examples include 18/7, 5/5, and 22/3.
-
Mixed Number: A mixed number combines a whole number and a proper fraction. A proper fraction has a numerator smaller than the denominator. Examples include 2 4/7, 1 1/2, and 3 1/3.
Converting an improper fraction to a mixed number essentially represents the fraction as a whole number plus a remaining fractional part. This representation often provides a more intuitive understanding of the quantity represented by the fraction.
Method 1: Long Division
The most common and straightforward method for converting an improper fraction to a mixed number involves long division. Let's use 18/7 as our example:
-
Divide the numerator by the denominator: Divide 18 (the numerator) by 7 (the denominator).
18 ÷ 7 = 2 with a remainder of 4
-
The quotient becomes the whole number: The quotient (the result of the division) is 2. This will be the whole number part of our mixed number.
-
The remainder becomes the numerator of the proper fraction: The remainder is 4. This becomes the numerator of the fraction part of our mixed number.
-
The denominator remains the same: The denominator stays the same (7).
-
Combine the whole number and the fraction: Therefore, 18/7 as a mixed number is 2 4/7.
Method 2: Repeated Subtraction
This method is conceptually useful, particularly for visualizing the process. It involves repeatedly subtracting the denominator from the numerator until the result is less than the denominator:
-
Subtract the denominator from the numerator: Subtract 7 from 18: 18 - 7 = 11
-
Repeat the subtraction: Subtract 7 from the result: 11 - 7 = 4
-
Count the number of subtractions: We subtracted 7 twice (2 times). This is the whole number part of our mixed number.
-
The final result is the numerator of the fraction: The remaining 4 is the numerator of our fraction.
-
The denominator remains the same: The denominator remains 7.
-
Combine: The mixed number is again 2 4/7.
Why Convert Improper Fractions to Mixed Numbers?
Converting improper fractions to mixed numbers offers several advantages:
-
Improved understanding: Mixed numbers often provide a clearer and more intuitive representation of a quantity. For instance, understanding that 18/7 represents two full units and an additional 4/7 is easier to grasp than the improper fraction alone.
-
Easier comparisons: Comparing mixed numbers is often easier than comparing improper fractions. For example, comparing 2 4/7 to 3 1/7 is simpler than comparing 18/7 to 22/7.
-
Simplified calculations: In some calculations, especially those involving addition or subtraction, working with mixed numbers can be more efficient.
-
Real-world applications: Mixed numbers are commonly used in everyday life, such as measuring ingredients in recipes or calculating distances. For example, saying you need 2 1/2 cups of flour is much clearer than saying you need 5/2 cups of flour.
Practicing with Different Improper Fractions
Let's practice converting a few more improper fractions to mixed numbers using the long division method:
-
25/4: 25 ÷ 4 = 6 with a remainder of 1. Therefore, 25/4 = 6 1/4.
-
31/6: 31 ÷ 6 = 5 with a remainder of 1. Therefore, 31/6 = 5 1/6.
-
17/5: 17 ÷ 5 = 3 with a remainder of 2. Therefore, 17/5 = 3 2/5.
-
47/9: 47 ÷ 9 = 5 with a remainder of 2. Therefore, 47/9 = 5 2/9.
-
100/11: 100 ÷ 11 = 9 with a remainder of 1. Therefore, 100/11 = 9 1/11.
Converting Mixed Numbers back to Improper Fractions
The reverse process is also crucial. To convert a mixed number back to an improper fraction:
-
Multiply the whole number by the denominator: For example, in 2 4/7, multiply 2 by 7 = 14.
-
Add the numerator: Add the result (14) to the numerator (4): 14 + 4 = 18.
-
Keep the denominator the same: The denominator remains 7.
-
The result is the improper fraction: The improper fraction is 18/7.
Applications in Real-World Scenarios
The conversion between improper fractions and mixed numbers is vital in numerous practical scenarios:
-
Baking and Cooking: Recipes frequently use mixed numbers to specify ingredient quantities (e.g., 1 1/2 cups of sugar).
-
Construction and Engineering: Measurements in construction and engineering often involve mixed numbers to express precise dimensions.
-
Timekeeping: Time is often expressed using mixed numbers (e.g., 2 1/2 hours).
-
Data Analysis: Data analysis might involve using fractions, and representing them as mixed numbers can improve readability and interpretation.
-
Finance: Calculations involving shares or portions of a whole often utilize fractions and mixed numbers.
Advanced Concepts and Further Exploration
While this guide focuses on the fundamentals, several advanced concepts build upon this foundational knowledge:
-
Working with negative fractions: The same principles apply when dealing with negative improper fractions and mixed numbers.
-
Adding and subtracting mixed numbers: Efficient methods exist for adding and subtracting mixed numbers without converting them back to improper fractions.
-
Multiplying and dividing mixed numbers: Specific procedures facilitate efficient multiplication and division with mixed numbers.
-
Fractions and decimals: Understanding the relationship between fractions, mixed numbers, and decimals is important for broader mathematical fluency.
Conclusion
Mastering the conversion between improper fractions and mixed numbers is a cornerstone of arithmetic. Understanding the different methods, their applications, and their relevance to real-world scenarios is crucial for anyone seeking to improve their mathematical skills. Practice regularly, explore different examples, and don't hesitate to delve into the more advanced topics to further strengthen your understanding. Remember, consistent practice is key to achieving mastery. By applying the techniques outlined in this comprehensive guide, you’ll gain confidence in working with fractions and improve your problem-solving abilities in various mathematical situations.
Latest Posts
Latest Posts
-
Identify The Phases Of The Eukaryotic Cell Cycle
May 13, 2025
-
2 Gallons Is How Many Liters
May 13, 2025
-
What Temp Does Water Boil Kelvin
May 13, 2025
-
An Object At Rest May Have
May 13, 2025
-
1 Us Dollar Is Equal To How Many Cents
May 13, 2025
Related Post
Thank you for visiting our website which covers about 18 7 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.