123 Divided By 13 With Remainder
Juapaving
May 12, 2025 · 5 min read
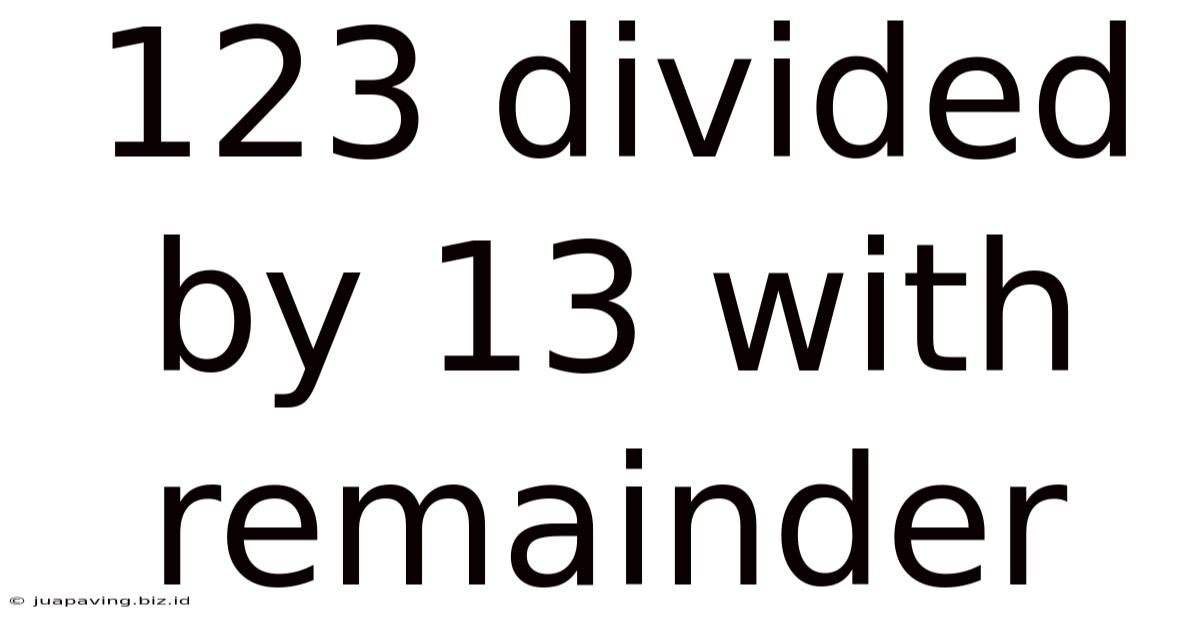
Table of Contents
123 Divided by 13: A Deep Dive into Division with Remainders
The seemingly simple question of "123 divided by 13 with remainder" opens a door to a fascinating exploration of fundamental mathematical concepts. While the answer itself is straightforward, understanding the process and its implications within broader mathematical contexts reveals a wealth of knowledge. This article will delve into this seemingly simple division problem, examining the process, exploring different methods of solving it, and demonstrating its relevance in various applications.
Understanding Division with Remainders
Division, at its core, is the process of splitting a quantity into equal parts. When we divide 123 by 13, we're asking how many times 13 can fit completely into 123. However, in many instances, a complete division isn't possible; there's a leftover amount, known as the remainder. This remainder signifies the portion of the original number that is "left over" after the equal division.
The standard form for representing division with a remainder is:
Dividend = (Divisor × Quotient) + Remainder
In our example:
- Dividend: 123 (the number being divided)
- Divisor: 13 (the number we are dividing by)
- Quotient: The result of the division (how many times 13 fits into 123)
- Remainder: The amount left over after the division
Methods for Calculating 123 Divided by 13
Several methods can determine the quotient and remainder when dividing 123 by 13. Let's explore a few:
1. Long Division
Long division is a classic algorithm for performing division. It systematically breaks down the division process into smaller, manageable steps.
9
13 | 123
-117
6
Following the long division process, we find that 13 goes into 123 nine times (the quotient) with a remainder of 6. Therefore, 123 divided by 13 is 9 with a remainder of 6.
2. Repeated Subtraction
This method involves repeatedly subtracting the divisor (13) from the dividend (123) until the result is less than the divisor. The number of subtractions represents the quotient, and the final result is the remainder.
123 - 13 = 110 110 - 13 = 97 97 - 13 = 84 84 - 13 = 71 71 - 13 = 58 58 - 13 = 45 45 - 13 = 32 32 - 13 = 19 19 - 13 = 6
We subtracted 13 nine times before reaching a number less than 13. Thus, the quotient is 9, and the remainder is 6.
3. Using Multiplication Tables
A strong understanding of multiplication tables can facilitate mental calculation. We can reason that 13 multiplied by 10 is 130, which is slightly more than 123. Trying smaller multiples, we find that 13 multiplied by 9 is 117. Subtracting 117 from 123 gives us a remainder of 6.
Applications of Division with Remainders
The concept of division with remainders extends far beyond basic arithmetic. It finds practical applications in numerous fields:
1. Everyday Life Scenarios
- Sharing Items: Imagine you have 123 candies to distribute evenly among 13 friends. Each friend would receive 9 candies (the quotient), and you'd have 6 candies left over (the remainder).
- Grouping Objects: If you need to arrange 123 books into stacks of 13, you'll have 9 full stacks and 6 books remaining.
2. Computer Science
- Modular Arithmetic: Remainders play a crucial role in modular arithmetic, a branch of number theory extensively used in cryptography and computer science. The modulo operator (%) returns the remainder of a division. For example, 123 % 13 = 6. This is fundamental in hash tables, random number generation, and many other computational processes.
- Data Structures: Remainders can be used in techniques like hashing to efficiently organize and access data in computer systems.
3. Engineering and Design
- Material Allocation: In engineering projects, calculating remainders helps determine the optimal use of resources, ensuring minimal waste. For instance, if you're cutting 123 meters of fabric into 13-meter pieces, you'll need to account for the leftover 6 meters.
4. Time and Measurement
- Converting Units: Remainders are essential when converting units of time or measurement. For example, converting 123 minutes into hours and minutes would result in 2 hours and 3 minutes (123/60 = 2 with a remainder of 3).
Expanding on the Concept: Exploring Divisibility Rules
Understanding divisibility rules enhances our ability to quickly determine whether a number is divisible by another without performing long division. While there isn't a specific divisibility rule for 13, understanding divisibility rules for other numbers provides insight into number theory and improves mental math skills.
Divisibility rules for some common numbers include:
- Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, or 8).
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3.
- Divisibility by 4: A number is divisible by 4 if its last two digits are divisible by 4.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 6: A number is divisible by 6 if it's divisible by both 2 and 3.
- Divisibility by 9: A number is divisible by 9 if the sum of its digits is divisible by 9.
- Divisibility by 10: A number is divisible by 10 if its last digit is 0.
While these rules don't directly solve 123 divided by 13, they illustrate the underlying principles of divisibility and number theory which are closely related to the concept of remainders.
Conclusion: The Significance of a Simple Division Problem
The seemingly trivial problem of 123 divided by 13 reveals a deeper understanding of fundamental mathematical concepts. From the straightforward application of long division to the broader implications in various fields, this simple problem highlights the importance of division with remainders in everyday life and advanced applications. Mastering this concept forms a solid foundation for further exploration in mathematics, computer science, and other quantitative disciplines. The ability to efficiently and accurately calculate remainders enhances problem-solving skills and contributes to a more comprehensive understanding of the numerical world around us. The exploration of divisibility rules further enriches this understanding, empowering us with efficient mental calculation strategies and deepening our appreciation for the elegance and interconnectedness of mathematical principles.
Latest Posts
Related Post
Thank you for visiting our website which covers about 123 Divided By 13 With Remainder . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.