12.5 Percent As A Fraction In Simplest Form
Juapaving
May 13, 2025 · 4 min read
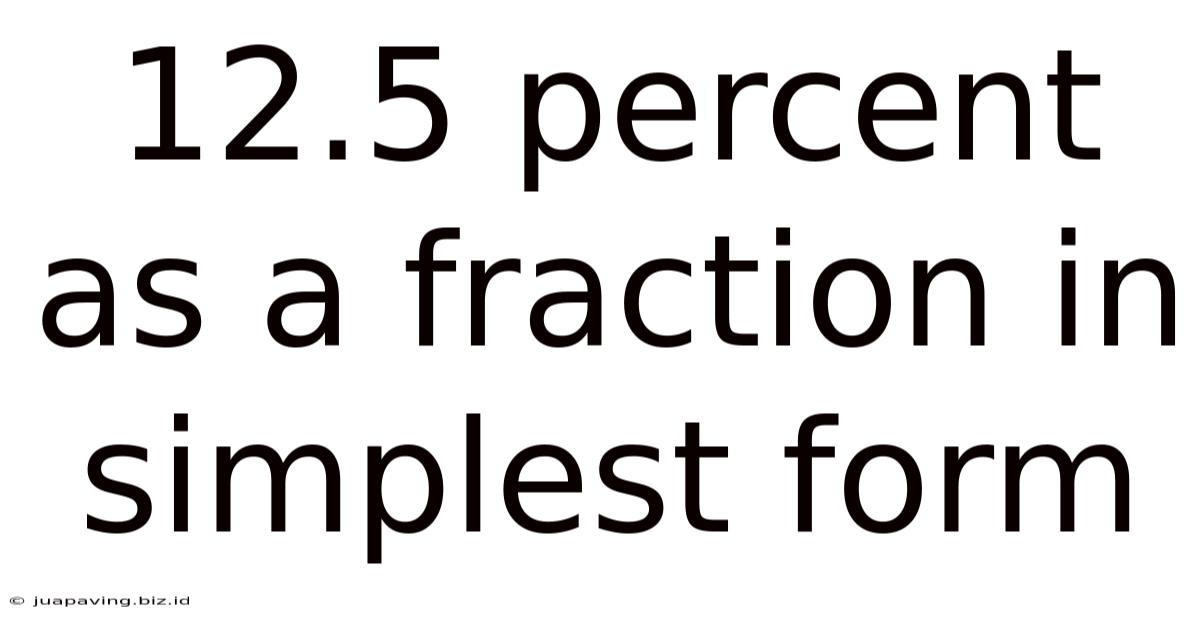
Table of Contents
12.5 Percent as a Fraction in Simplest Form: A Comprehensive Guide
Understanding percentages and their fractional equivalents is a fundamental skill in mathematics with wide-ranging applications in various fields, from finance and accounting to everyday calculations. This comprehensive guide delves into the process of converting 12.5 percent into its simplest fractional form, providing a detailed explanation and exploring related concepts to enhance your understanding.
Understanding Percentages and Fractions
Before we dive into the conversion, let's review the basic concepts. A percentage represents a fraction of 100. The word "percent" literally means "per hundred." A fraction, on the other hand, expresses a part of a whole, represented by a numerator (the top number) and a denominator (the bottom number).
For example, 50% means 50 out of 100, which can be written as the fraction 50/100. This fraction can be further simplified by dividing both the numerator and the denominator by their greatest common divisor (GCD), which in this case is 50. This simplifies the fraction to 1/2.
Converting 12.5 Percent to a Fraction
To convert 12.5% to a fraction, we follow these steps:
Step 1: Write the percentage as a fraction with a denominator of 100.
12.5% can be written as 12.5/100.
Step 2: Eliminate the decimal point in the numerator.
To do this, we multiply both the numerator and the denominator by 10 (since there's one digit after the decimal point). This gives us:
(12.5 x 10) / (100 x 10) = 125/1000
Step 3: Simplify the fraction.
Now we need to find the greatest common divisor (GCD) of 125 and 1000 to simplify the fraction to its lowest terms. The GCD of 125 and 1000 is 125. Dividing both the numerator and the denominator by 125, we get:
125 ÷ 125 = 1 1000 ÷ 125 = 8
Therefore, the simplest form of the fraction is 1/8.
Alternative Method: Using Decimal Conversion
Another approach involves converting the percentage directly to a decimal first and then to a fraction.
Step 1: Convert the percentage to a decimal.
To convert a percentage to a decimal, divide the percentage by 100. 12.5% divided by 100 is 0.125.
Step 2: Convert the decimal to a fraction.
0.125 can be written as 125/1000. As we saw in the previous method, this fraction simplifies to 1/8.
Understanding the Simplest Form
The concept of "simplest form" in fractions is crucial. A fraction is in its simplest form when the numerator and denominator have no common factors other than 1. This means that the fraction cannot be further reduced. Simplifying fractions makes them easier to understand and work with.
For instance, while 125/1000 is a correct representation of 12.5%, it's not the most efficient or easily understandable form. 1/8 is a much simpler and more concise way to represent the same value.
Applications of Fraction and Percentage Conversions
The ability to convert between percentages and fractions is essential in many real-world scenarios. Here are a few examples:
Finance and Accounting
- Calculating interest: Interest rates are often expressed as percentages, but calculations often require converting them into fractions.
- Determining discounts: Sales discounts are usually given as percentages, but calculating the actual discount amount requires converting the percentage to a fraction.
- Analyzing financial statements: Financial ratios and analyses frequently involve working with fractions and percentages.
Everyday Calculations
- Calculating tips: Determining a tip amount at a restaurant often involves using percentages.
- Measuring ingredients: Recipes often list ingredients as fractions or percentages of the total.
- Understanding statistics: Statistics frequently present data as percentages which might require conversion to fractions for deeper analysis.
Advanced Mathematical Applications
- Probability: Probabilities are often expressed as fractions, and understanding percentage equivalents is helpful.
- Algebra: Solving equations may involve converting percentages to fractions.
- Calculus: Certain calculus problems may require working with fractions and percentages.
Practice Problems
To solidify your understanding, try converting the following percentages to fractions in their simplest form:
- 25%
- 75%
- 37.5%
- 62.5%
- 87.5%
Solutions:
- 25% = 25/100 = 1/4
- 75% = 75/100 = 3/4
- 37.5% = 375/1000 = 3/8
- 62.5% = 625/1000 = 5/8
- 87.5% = 875/1000 = 7/8
Conclusion
Converting 12.5% to its simplest fractional form, 1/8, is a straightforward process that demonstrates the fundamental relationship between percentages and fractions. Mastering this conversion is crucial for various applications in mathematics, finance, and everyday life. Understanding the process and practicing with different percentages will improve your mathematical skills and problem-solving abilities. Remember that simplifying fractions to their lowest terms is important for clarity and efficiency in calculations. This guide provides a comprehensive approach, detailing steps and alternative methods, ensuring a thorough understanding of this essential concept. Regular practice will enhance your fluency in working with percentages and fractions.
Latest Posts
Latest Posts
-
How Much Is 36 In In Feet
May 13, 2025
-
Words With The Letter Z And A
May 13, 2025
-
Select The Correct Iupac Name For The Compound
May 13, 2025
-
Pepsinogen Is Secreted By What Cells
May 13, 2025
-
What Is The Bond Angle Of O3
May 13, 2025
Related Post
Thank you for visiting our website which covers about 12.5 Percent As A Fraction In Simplest Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.